On Pseudo-Differential Operators

Fourier analysis can be used to understand more complicated questions. For example, the relation of a function values to its normal derivative values on the boundary. Some relations between the quantities of interest may involve differential operators. Other relations may involve a more general class of operators, called pseudo-differential operators, which we briefly discuss here.

We consider elementary ideas from the theory of pseudo-differential operators which we demonstrate using some simple examples.

Example III Let $\phi$ be the solution of the equation

$$\begin{array}{ll} \Delta \phi = 0 & \Omega \\ \frac{\partial \phi}{\partial n} = \alpha & \partial \Omega, \end{array}$$ (13)

where the domain $\Omega$ is one of the two cases

$$\begin{array}{l} \Omega _2 = \{ (x,z) \vert z > 0 \} \\ \Omega _3 = \{ (x,y,z ) \vert z > 0 \}, \end{array}$$ (14)

and $\frac{\partial}{\partial n}$ is the outward normal derivative at the boundary.

One of the questions we need to answer with regard to shape optimization or boundary control problems is the nature of the mappings between the control variable $\alpha$, which in this case is a function defined on the boundary, and say the value of the solution on the boundary. That mapping is of course complicated and in general depends on the shape of the domain $\Omega$. However, high frequencies in $\alpha$ have only local effect on the solution $\phi$ (this is a general property for elliptic equations which we exploit ) and can be studied using Fourier techniques. We take

$$\alpha(x) = \exp ( i {\bf k} \cdot {\bf x})$$ (15)

where in case $\Omega = \Omega _3$ we take ${\bf k} = (k_1, k_2)$ and ${\bf x} = (x,y)$, and for $\Omega = \Omega _2$ we take ${\bf k} = k, {\bf x} = x$. Then $\phi$ is given by

$$\phi ({\bf x},z) = A \exp ( i {\bf k} \cdot {\bf x}) \exp (\sigma z).$$ (16)

A substitution of this expression for $\phi$ into the interior equation in (13) implies the following equation for $\sigma$

$$\sigma ^2 - \vert {\bf k } \vert ^2 = 0,$$ (17)

and there are two solution

$$\phi _1({\bf x},z) = A \exp ( i {\bf k} \cdot {\bf x} ) \exp... ... \exp ( i {\bf k} \cdot {\bf x} ) \exp ( \vert{\bf k}\vert z )$$ (18)

We look for a bounded solution in $\Omega$ and that is the one with $\exp ( - \vert k\vert z )$. The above expression for $\phi$ satisfies the interior equations in (13) for any value of $A$, but only one value will also satisfy the boundary condition. Substituting (18) into that boundary condition, gives

$$A = \frac{1}{\vert{\bf k}\vert}.$$ (19)

The coefficient $A$ that we have just found gives a very important information. It describes the mapping $T$ between $\alpha$ and the values of $\phi$ on the boundary,

$$\phi \vert _{\partial \Omega} = T \alpha.$$ (20)

What we have just found is a description of the mapping $T$ in the Fourier space, i.e., the symbol of $T$,

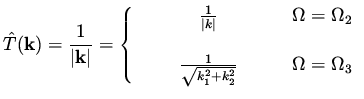$$\hat{T} ({\bf k}) = \frac{1}{\vert{\bf k}\vert} = \left\{ \begin{... ...{3cm} \\ \frac{1}{\sqrt{k_1^2 + k_2^2}}& \Omega = \Omega _3 \end{array}\right.$$ (21)

A Remark: For a general domain $\Omega$ we consider a small vicinity of a boundary point $x \in \partial \Omega$, and if the boundary is smooth at that point, we can flatten this piece of boundary by a proper transformation. We end up with a problem in half space, of the same form as above. The relation between $\alpha$ on the boundary, which is flat now, and $\phi$ inside the domain can be easily calculated using Fourier analysis.