|
Department of Mathematical Sciences
Events
People
Colloquia and Seminars
Conferences
Centers
Positions
Areas of Research
About the Department
Alumni |
Algorithms, Combinatorics and Optimization Seminar
Rutgers and IAS Title: The minimum number of nonnegative edges in hypergraphs Abstract: An r-unform n-vertex hypergraph H is said to have the Manickam–Miklós–Singhi (MMS) property if for every assignment of weights to its vertices with nonnegative sum, the number of edges whose total weight is nonnegative is at least the minimum degree of H. In this talk I will show that for n>10r3, every r-uniform n-vertex hypergraph with equal codegrees has the MMS property, and the bound on n is essentially tight up to a constant factor. An immediate corollary of this result is the vector space Manickam–Miklós–Singhi conjecture which states that for n ≥ 4k and any weighting on the 1-dimensional subspaces of Fqn with nonnegative sum, the number of nonnegative k-dimensional subspaces is at least 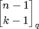 . I will also discuss two additional generalizations, which can be regarded as analogues of the Erdős–Ko–Rado theorem on k-intersecting families.Joint work with Benny Sudakov. |
