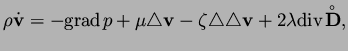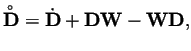Abstract
The direct numerical simulation of
turbulence at Reynolds numbers in excess of a few thousand provides a
formidable computational problem, even with access to state-of-the-
art supercomputers. For this reason, there remains a strong interest
in alternative methods that resolve only large-scale motions while
modeling small-scale motions via filtering. The most well-known
approaches are large eddy simulation and closure approximations based
on Reynolds averaged equations. While these methods reduce
computational costs, the additional dissipation associated with
filtering can lead to artificially sluggish flows. A method that
avoids this difficulty is provided by simulations based on an equation
-- the Navier- Stokes-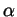 equation -- obtained by Lagrangian
averaging. Known as the Navier- Stokes-
equation -- obtained by Lagrangian
averaging. Known as the Navier- Stokes-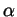 equation, that
equation has the form
equation, that
equation has the form
which
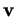
is the fluid velocity, subject to the incompressibility constraint
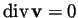
,
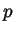
is the pressure,
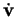
is the material time derivative
of
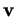
,
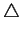
is the Laplace operator,
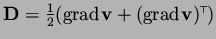
is the stretch-rate,
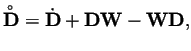
with
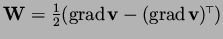 the spin, is the
corotational rate of
the spin, is the
corotational rate of 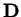 . Aside from the density
. Aside from the density 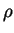 and the
shear viscosity
and the
shear viscosity 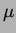 of the fluid, the Navier-Stokes-
of the fluid, the Navier-Stokes-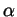 equation involves an additional material parameter
equation involves an additional material parameter 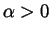 carrying dimensions of length. Within the framework of Lagrangian
averaging,
carrying dimensions of length. Within the framework of Lagrangian
averaging, 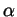 is the statistical correlation length of the
excursions taken by a fluid particle away from its phase-averaged
trajectory. More intuitively,
is the statistical correlation length of the
excursions taken by a fluid particle away from its phase-averaged
trajectory. More intuitively, 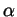 can be interpreted as the
characteristic linear dimension of the smallest eddies that the model
is capable of resolving. In this talk, we use the framework of Fried
& Gurtin (2005) to develop an alternative continuum-mechanical
formulation leading to a generalization of Navier-Stokes-
can be interpreted as the
characteristic linear dimension of the smallest eddies that the model
is capable of resolving. In this talk, we use the framework of Fried
& Gurtin (2005) to develop an alternative continuum-mechanical
formulation leading to a generalization of Navier-Stokes-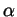 equation. That generalization involves not one but two additional
material length scales, one being of energetic origin and the other
being of dissipative origin. In contrast to Lagrangian averaging, our
formulation deliver boundary conditions and a complete thermodynamic
framework. The boundary condition also involve yet one more material
length scale. As an application, we consider the problem of classical
problem of turbulent flow in a plane, rectangular channel with fixed,
impermeable, slip-free walls and make comparisons with results
obtained from experiment and direct numerical simulations. An
interesting feature of our results is that when the additional
material parameter associated with the boundary conditions is signed
to ensure satisfaction of the second law at the channel walls the
theory delivers solutions that agree neither qualitatively nor
quantitavely with the experimentally and numerically observed
features of plane channel flow. On the contrary, we find excellent
agreement when the sign of the additional material parameter
associated with the boundary conditions violates the second
law. Although Marsden & Shkoller (2001) recently established
well-posedness results for the Navier-Stokes-
equation. That generalization involves not one but two additional
material length scales, one being of energetic origin and the other
being of dissipative origin. In contrast to Lagrangian averaging, our
formulation deliver boundary conditions and a complete thermodynamic
framework. The boundary condition also involve yet one more material
length scale. As an application, we consider the problem of classical
problem of turbulent flow in a plane, rectangular channel with fixed,
impermeable, slip-free walls and make comparisons with results
obtained from experiment and direct numerical simulations. An
interesting feature of our results is that when the additional
material parameter associated with the boundary conditions is signed
to ensure satisfaction of the second law at the channel walls the
theory delivers solutions that agree neither qualitatively nor
quantitavely with the experimentally and numerically observed
features of plane channel flow. On the contrary, we find excellent
agreement when the sign of the additional material parameter
associated with the boundary conditions violates the second
law. Although Marsden & Shkoller (2001) recently established
well-posedness results for the Navier-Stokes-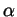 equation on
bounded domains, their analysis is based on thermodynamically stable
boundary conditions and therefore cannot pertain to turbulent
flows. The question of whether initial-boundary-value problems for
the Navier-Stokes-
equation on
bounded domains, their analysis is based on thermodynamically stable
boundary conditions and therefore cannot pertain to turbulent
flows. The question of whether initial-boundary-value problems for
the Navier-Stokes-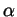 equations are well-posed when boundary
conditions appropriate to turbulence are imposed therefore remains
open. An additional question of central importance concerns whether
solutions to initial-boundary-value problems for the
Navier-Stokes-
equations are well-posed when boundary
conditions appropriate to turbulence are imposed therefore remains
open. An additional question of central importance concerns whether
solutions to initial-boundary-value problems for the
Navier-Stokes-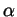 equations converge to solutions of
initial-boundary-value problems for the Navier-Stokes
equations. Because of the nonstandard thermodynamic structure of the
theory, it seems very likely that answers to the se questions will
require novel analytical approaches. This work is
equations converge to solutions of
initial-boundary-value problems for the Navier-Stokes
equations. Because of the nonstandard thermodynamic structure of the
theory, it seems very likely that answers to the se questions will
require novel analytical approaches. This work is
TUESDAY, November 15, 2005
Time: 1:30 P.M.
Location: