Monotonicity Properties of Ground States



Patricio Felmer
Universidad de Chile
Centro de Modelamiento Matematico
email: pfelmer@dim.uchile.cl



Abstract: It has been well-known that the scalar field equation

\begin{displaymath} \Delta u -u+u^p=0\quad\mbox{in}\quad R^N,\quad N\ge 3, \end{displaymath}

admits ground state solutions if and only if 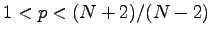$1<p<(N+2)/(N-2)$. For each fixed $p$ in this range, there corresponds a unique ground state (up to translation), as well as a unique positive solution to the Dirichlet boundary value problem

\begin{displaymath} \Delta u -u+u^p=0 \ \ \mbox{in} \ \ B, \ \ \mbox{and} \ \ u=0 \ \ \mbox{on} \ \ \partial B, \end{displaymath}

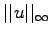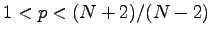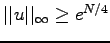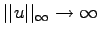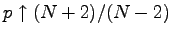for any finite ball $B$ in $R^N$. In this talk we show that the maximum value of such ground states, $\vert\vert u\vert\vert _\infty$, is an increasing function of $p$ for all $1<p<(N+2)/(N-2)$, with $\vert\vert u\vert\vert _\infty\ge e^{N/4}$, and $\vert\vert u\vert\vert _\infty\to \infty$ as $p\uparrow (N+2)/(N-2)$. Consequently, we derive a Liouville type theorem ensuring that there exists neither a ground state solution to this equation, nor a positive solution of the Dirichlet problem in any finite ball, with the maximum value less than $e^{N/4}$. Our proof relies on some fine analyses on the first variation of ground states with respect to $p$. 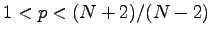The delicacy of this study can be evidenced by the fact that, on any fixed finite ball, the maximum value of positive solutions to the Dirichlet problem is never a monotone function of $p$ over the whole range $1<p<(N+2)/(N-2)$. We will also present some related problems and open questions.

The main result is obtained in collaboration with Quaas, Tang and Ye.